Appendix B — Slope and Deflection Tables

Slopes and Deflections of Simply Supported Beams
Beam Slope Max. Deflection Elastic Curve



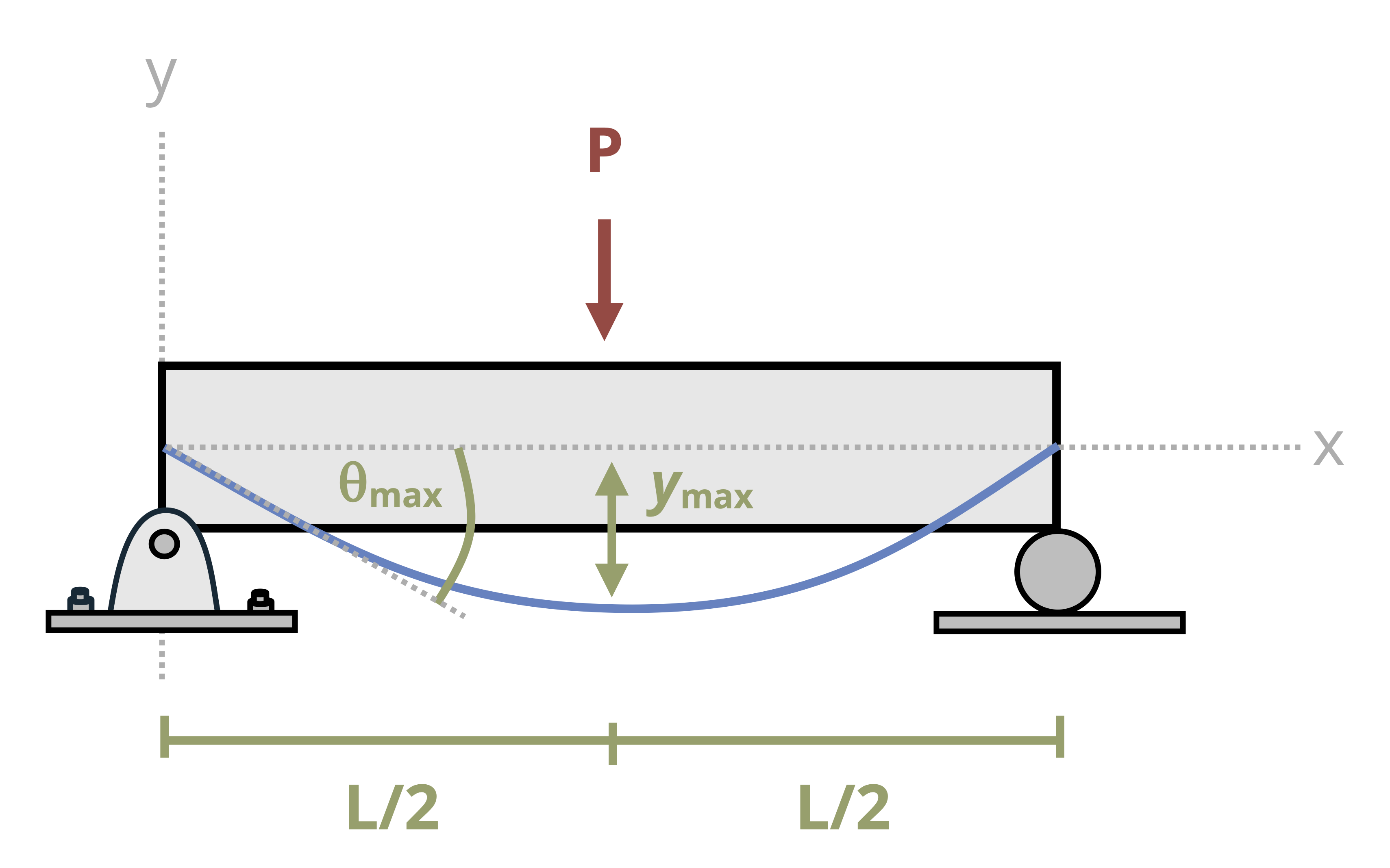\(\theta_{max}=- \frac{PL^2}{16EI}\)


\(y_{max}=-\frac{PL^3}{48EI}\)



\(y=-\frac{Px}{48EI}\left(3L^2-4x^2\right)\)

Valid for x ≤ L/2



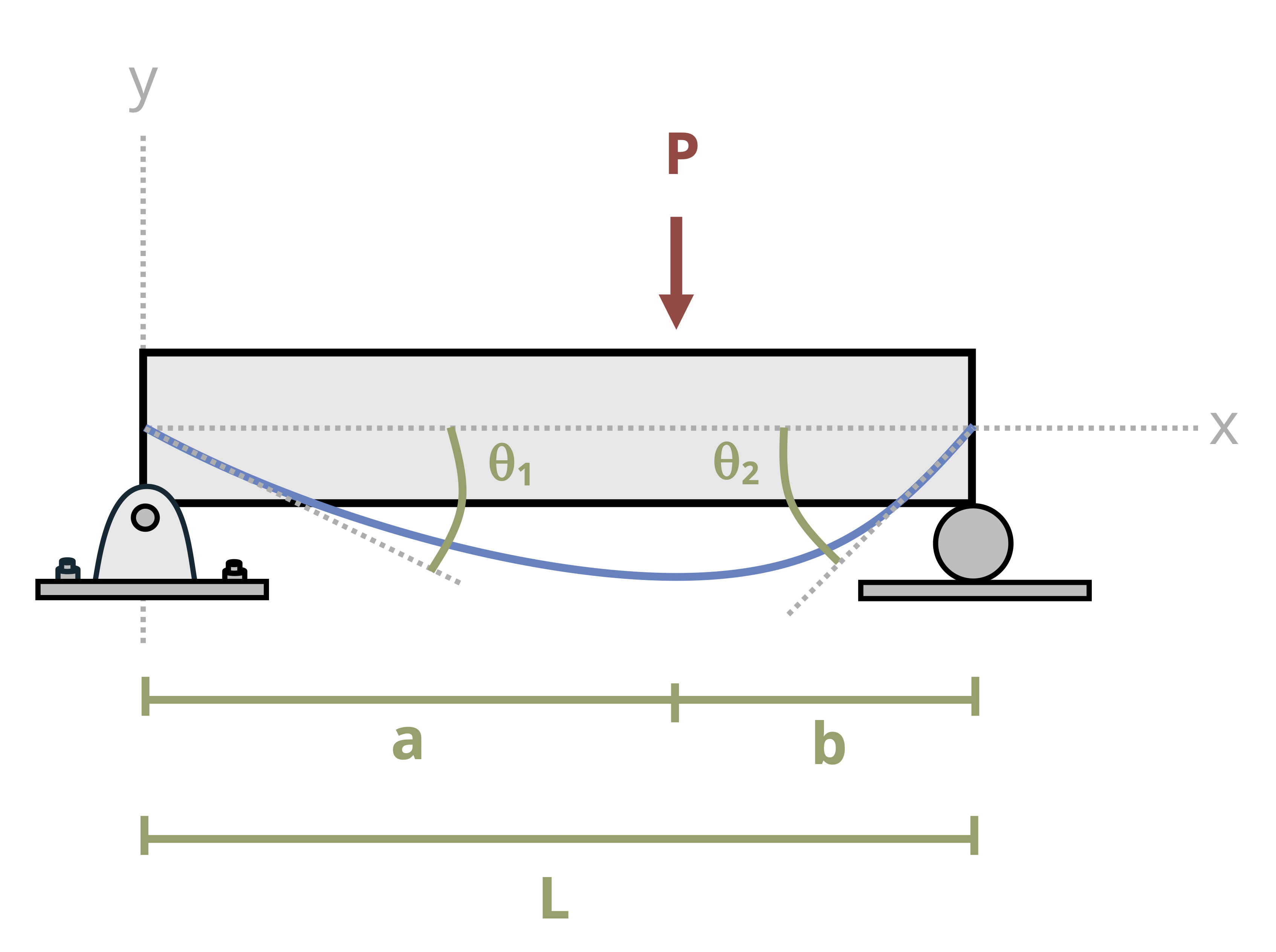\(\theta_A=-\frac{Pab(L+b)}{6EIL}\)

\(\theta_B=\frac{Pab(L+a)}{6EIL}\)



\(y_{max}=-\frac{Pb(L2-b2)^{3/2}}{9\sqrt{3}EIL}\)

At \(x=\sqrt{\frac{L^2-b^2}{3}}\)


\(y=-\frac{Pbx}{6EIL}(L^2-b^2-x^2)\)

Valid for x < a

At \(x=a,~~~y=-\frac{Pa^2b^2}{3EIL}\)



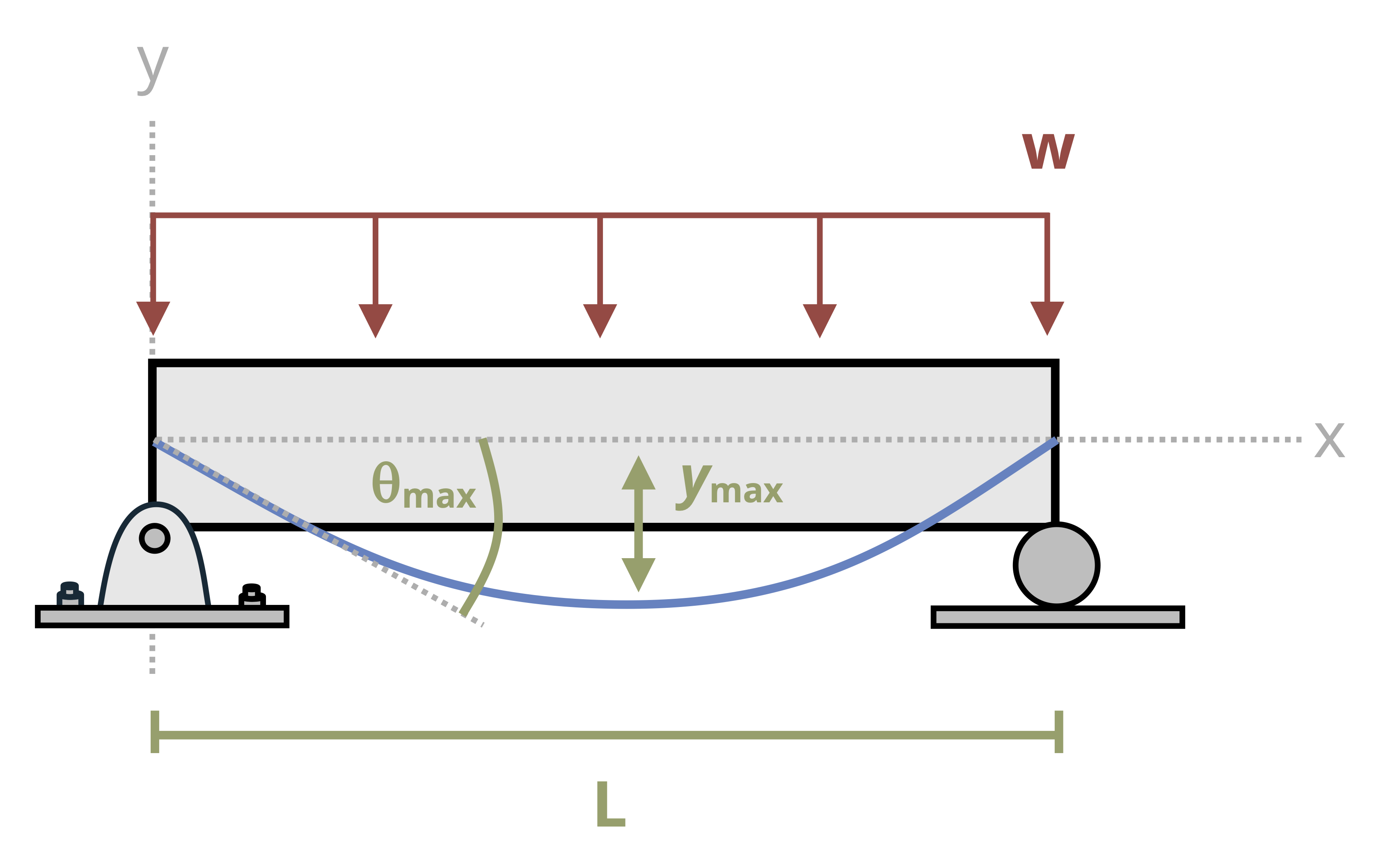\(\theta_{max}=-\frac{wL^3}{24EI}\)


\(y_{max}=-\frac{5wL^4}{384EI}\)


\(y=-\frac{wx}{24EI}(x^3-2Lx^2+L^3)\)



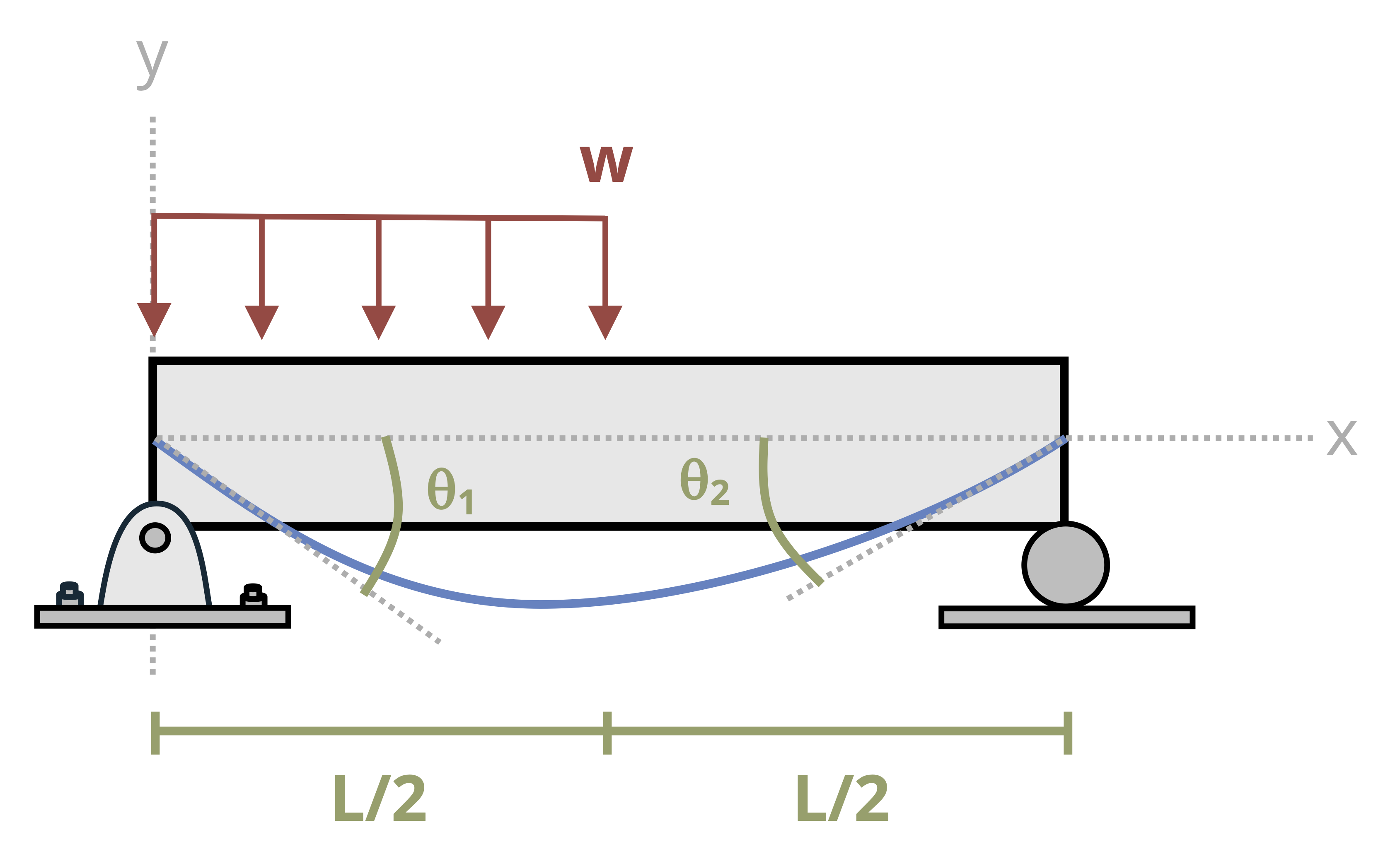\(\theta_A =-\frac{3wL^3}{128EI}\)

\(\theta_B =\frac{7wL^3}{384EI}\)



\(y_{max}=-\frac{wL^4}{152.37EI}\)

At \(x=0.4598L\)


\(y=-\frac{wx}{384EI}(16x^3-24Lx^2+9L^3)\)

Valid for \(0\le x\le\frac{L}{2}\)

At \(x=\frac{L}{2},~~~y=-\frac{5wL^4}{768EI}\)



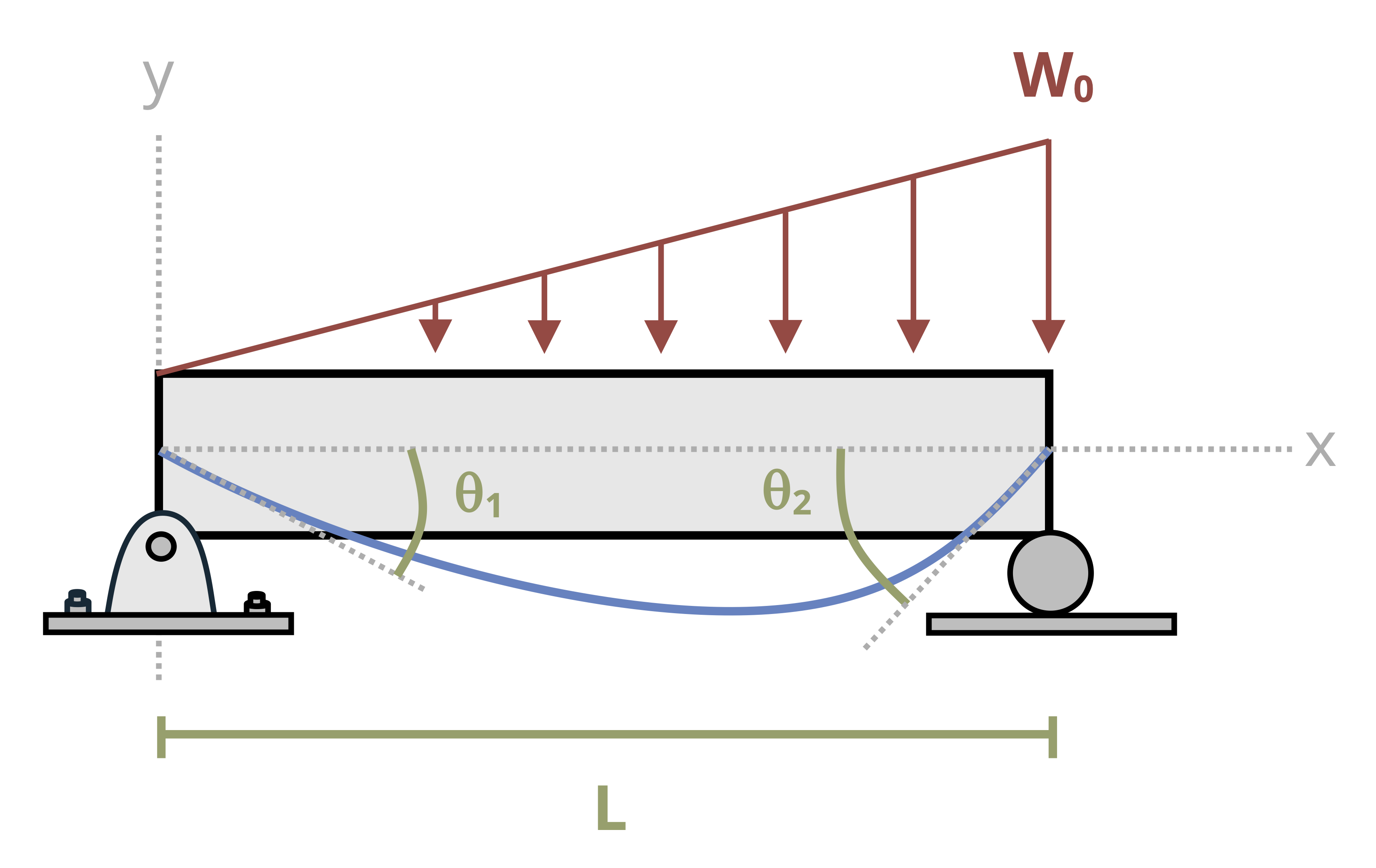\(\theta_A =-\frac{7wL^3}{360EI}\)

\(\theta_B =\frac{wL^3}{45EI}\)



\(y_{max}=-\frac{wL^4}{153.37EI}\)

At \(x=0.5193L\)


\(y=-\frac{wx}{360EIL}(3x^4-10L^2x^2+7L^4)\)


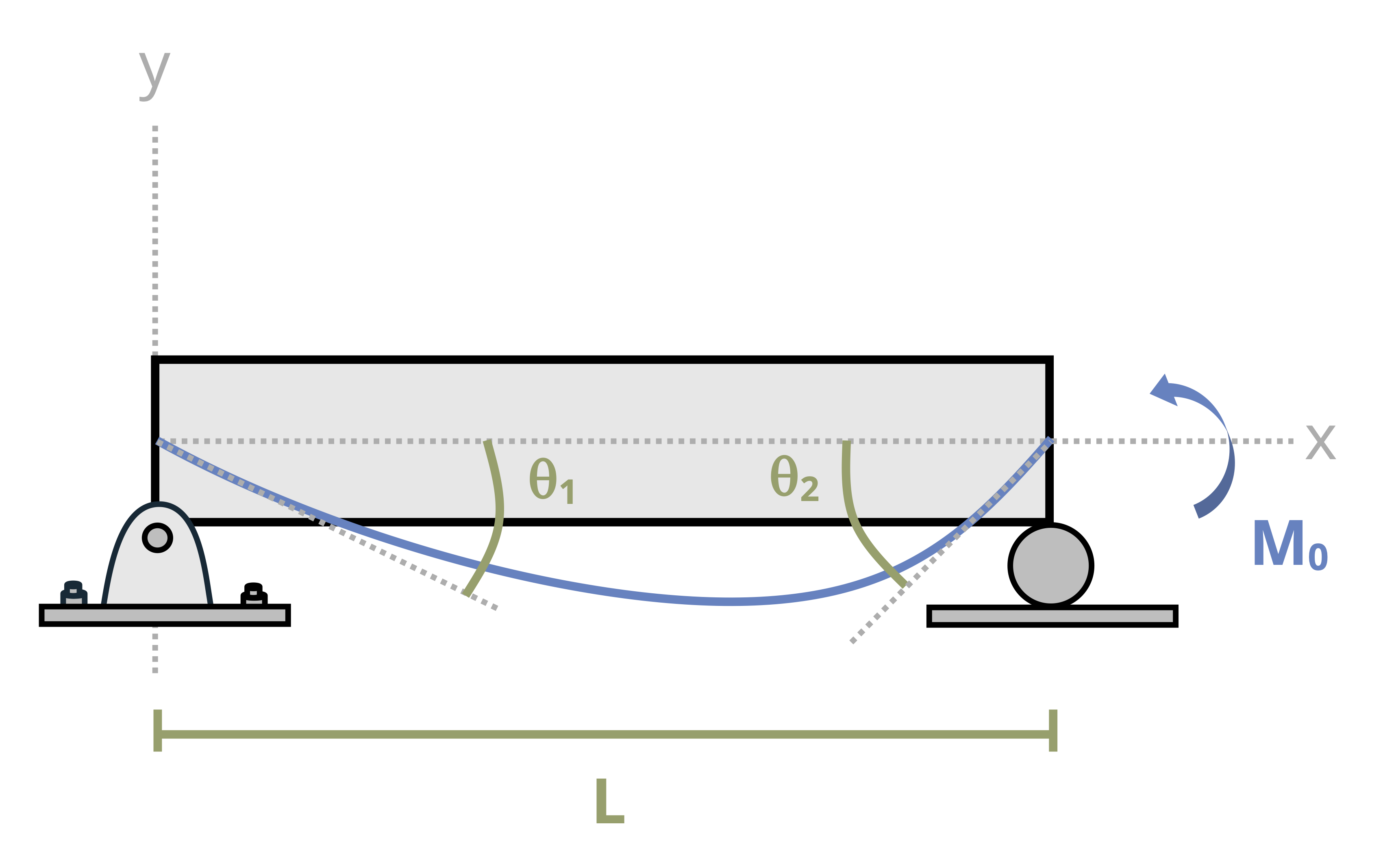\(\theta_A=-\frac{ML}{6EI}\)

\(\theta_B=\frac{ML}{3EI}\)



\(y_{max}=-\frac{ML^2}{9\sqrt{3}EI}\)

At \(x=\frac{L}{\sqrt{3}}\)


\(y=-\frac{Mx}{6EIL}(L^2-x^2)\)



Slopes and Deflections of Cantilever Beams
Beam Slope Max. Deflection Elastic Curve



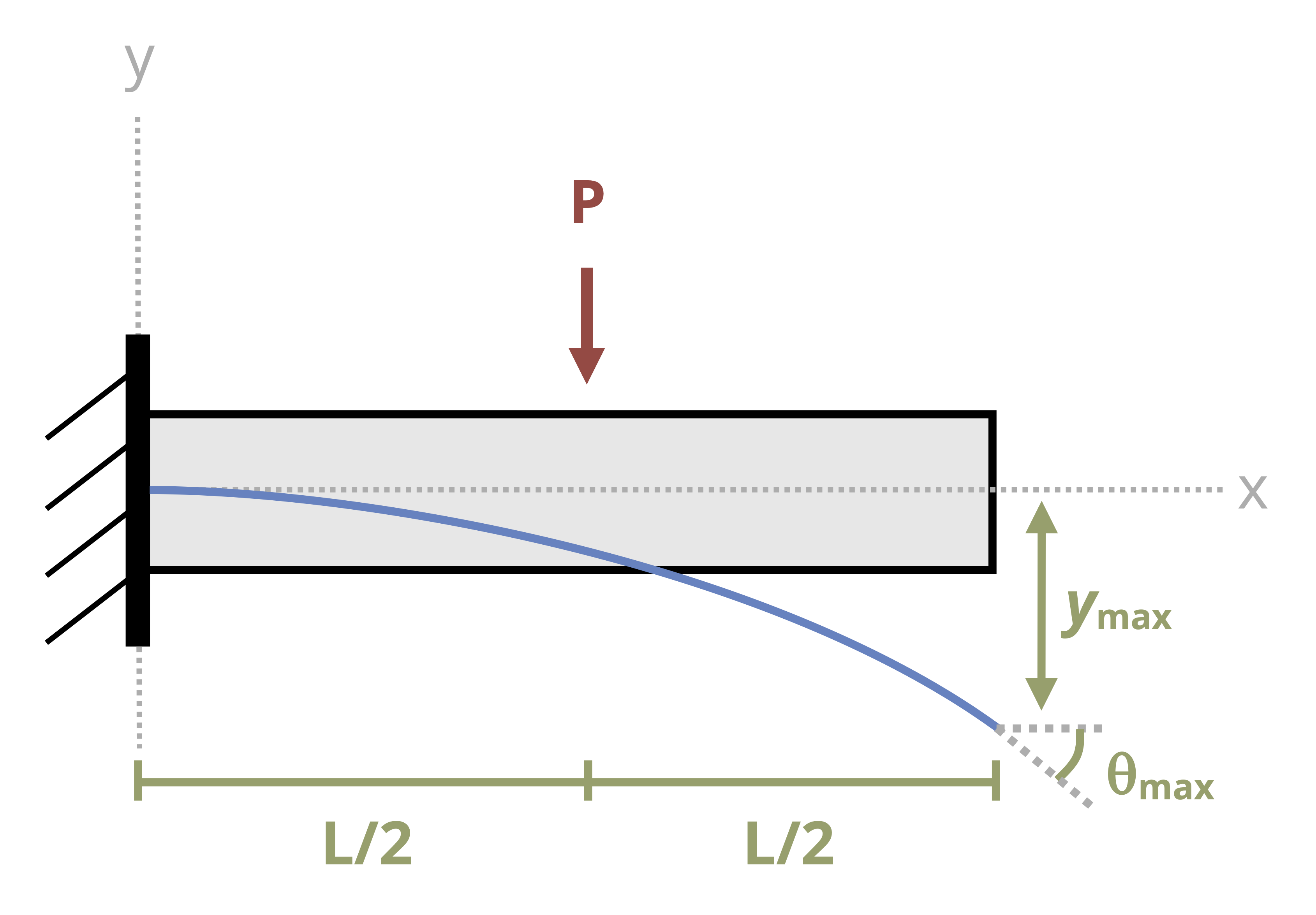
\(\theta_{max}=- \frac{PL^2}{2EI}\)


\(y_{max}=-\frac{PL^3}{3EI}\)


\(y = -\frac{Px^2}{6EI}(3L-x)\)



\(\theta_{max}=- \frac{PL^2}{8EI}\)


\(y_{max}=-\frac{5PL^3}{48EI}\)

\(y=-\frac{Px^2}{12EI}(3L-2x)\)

Valid for \(0\le x\le \frac{L}{2}\)

\(y=-\frac{PL^2}{48EI}(6x-L)\)

Valid for \(\frac{L}{2}\le x \le L\)



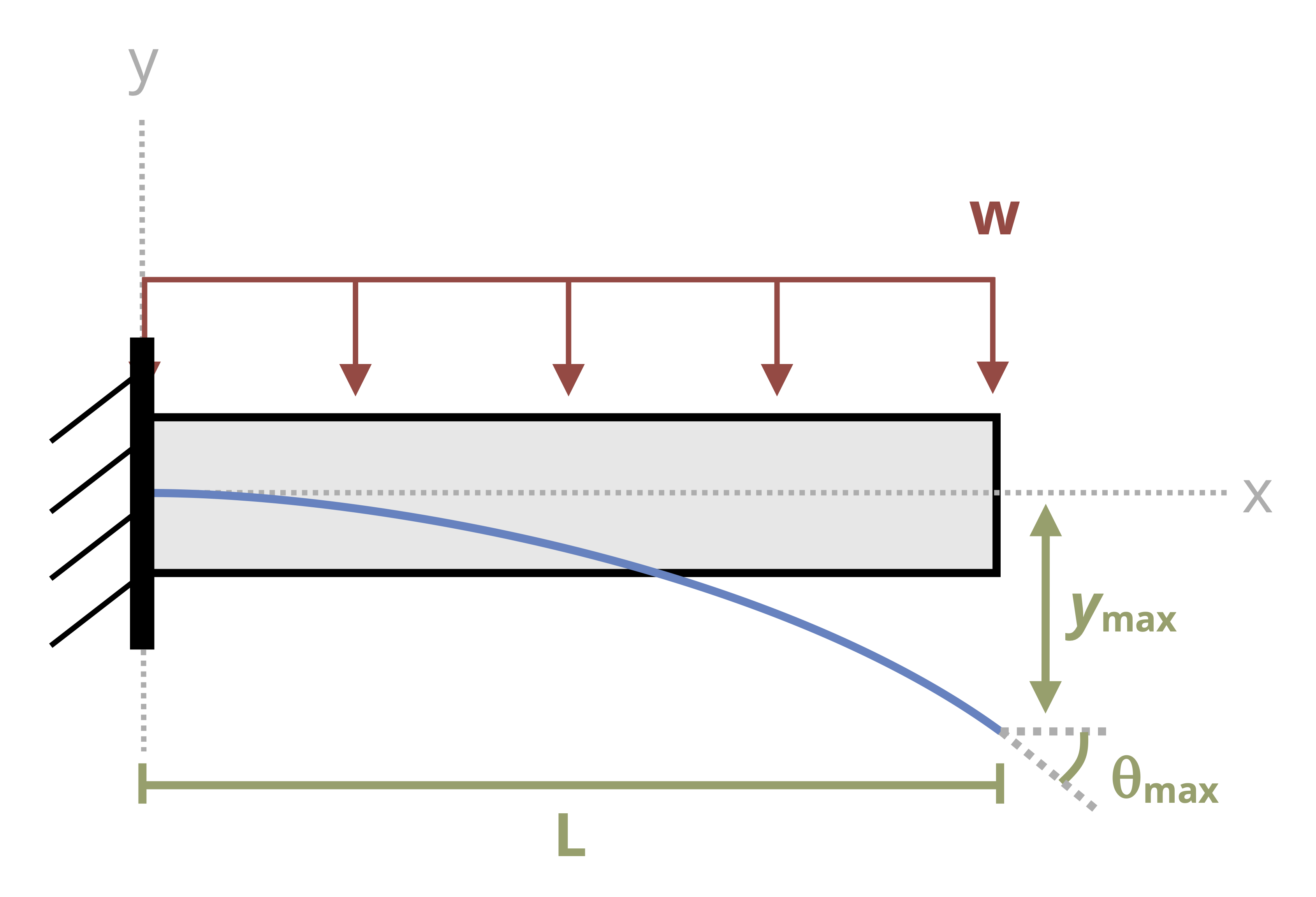\(\theta_{max}=- \frac{wL^3}{6EI}\)


\(y_{max}=-\frac{wL^4}{8EI}\)


\(y=-\frac{wx^2}{24EI}(x^2-4Lx+6L^2)\)



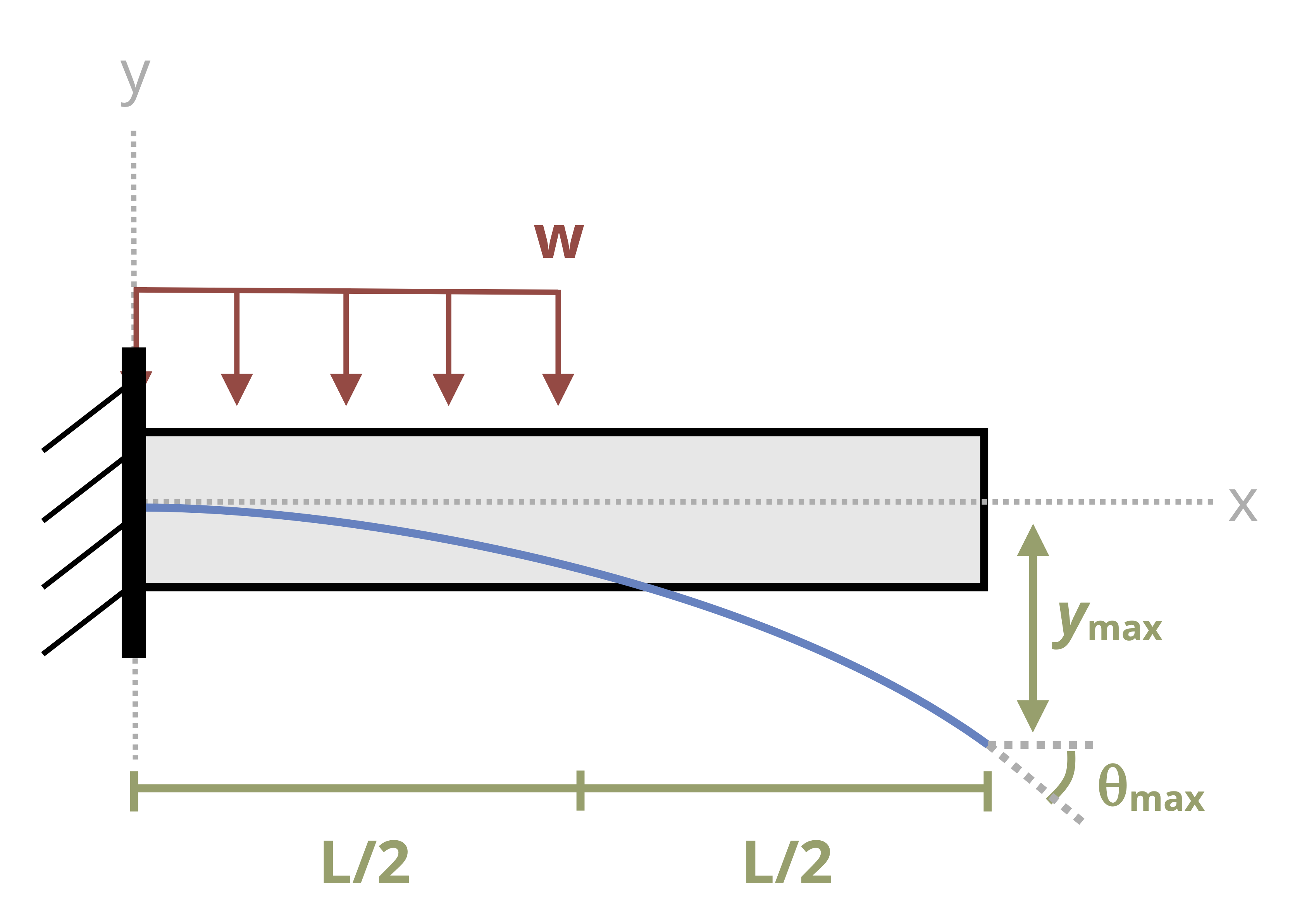\(\theta_{max}=-\frac{wL^3}{48EI}\)


\(y_{max}=-\frac{7wL^4}{384EI}\)

\(y=-\frac{wx^2}{24EI}(x^2-2Lx+1.5L^2)\)

Valid for \(0\le x\le \frac{L}{2}\)

\(y=-\frac{wL^3}{384EI}(8x-L)\)

Valid for \(\frac{L}{2}\le x\le L\)



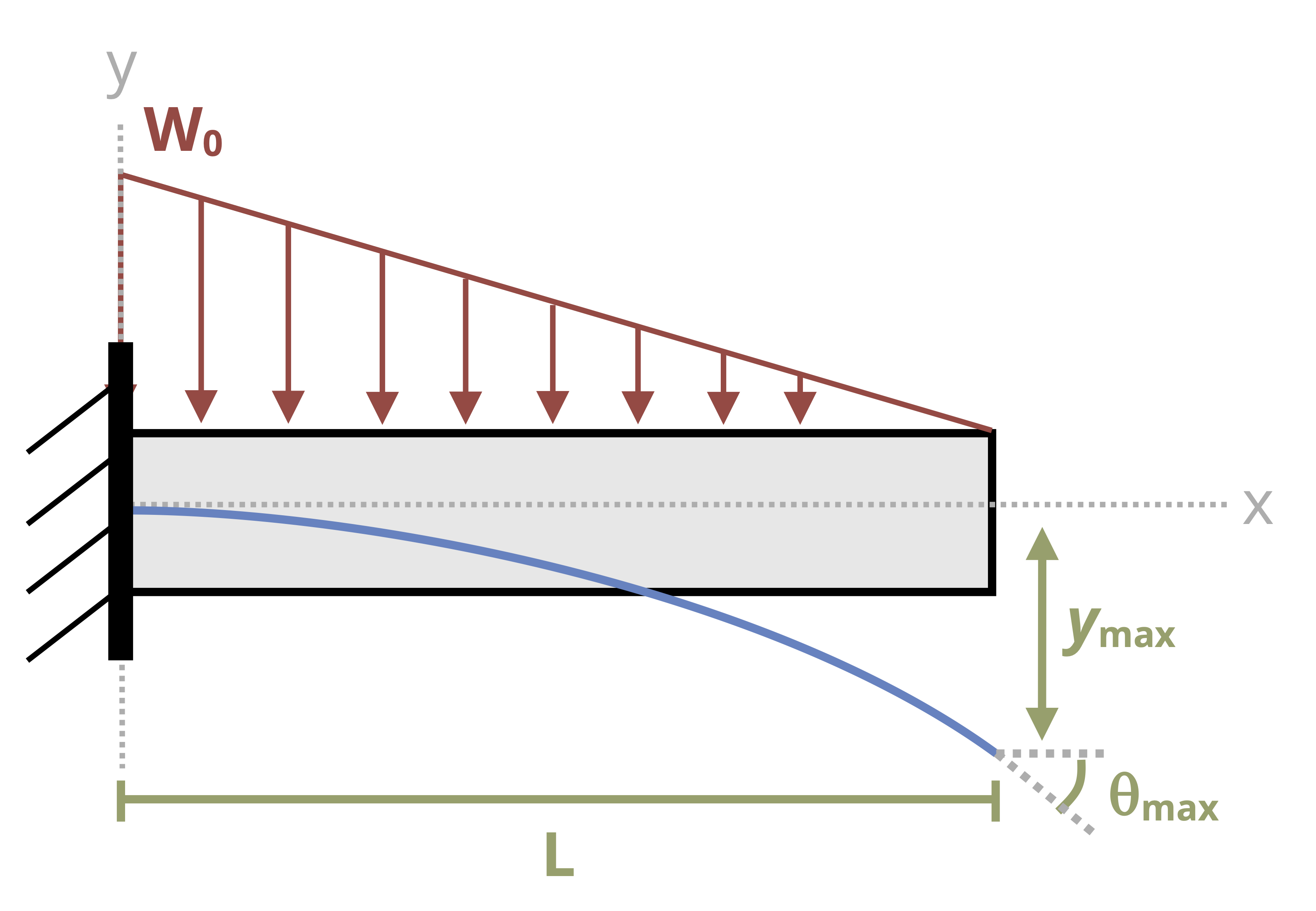\(\theta_{max}=-\frac{wL^3}{24EI}\)


\(y_{max}=-\frac{wL^4}{30EI}\)


\(y=-\frac{wx^2}{120EIL}(10L^3-10L^2x+5Lx^2-x^3)\)



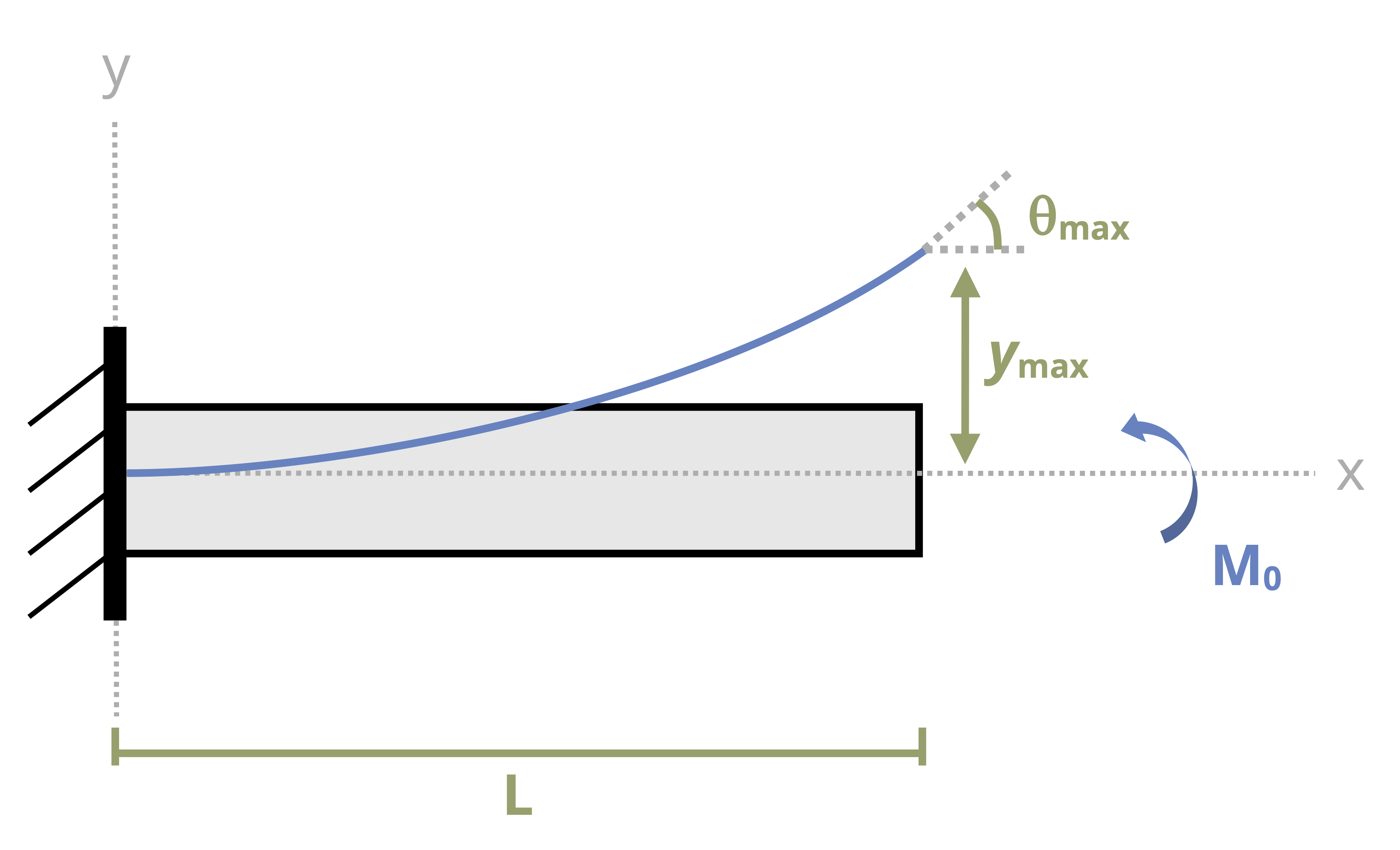\(\theta_{max}=\frac{ML}{EI}\)


\(y_{max}=\frac{ML^2}{2EI}\)


\(y=\frac{Mx^2}{2EI}\)

References

Click to expand

Figures

Appendix B figures: Kindred Grey. 2024. CC BY-NC-SA.